Lesson 43 covers:

• Elementary Level: Doubles Facts (2+2, 3+3, etc.)
• Mid Level: Adding and Subtracting Fractions with Like Denominators
• High Level: Solving One-Step Equations

Elementary Level (Kinder to Grade 2)

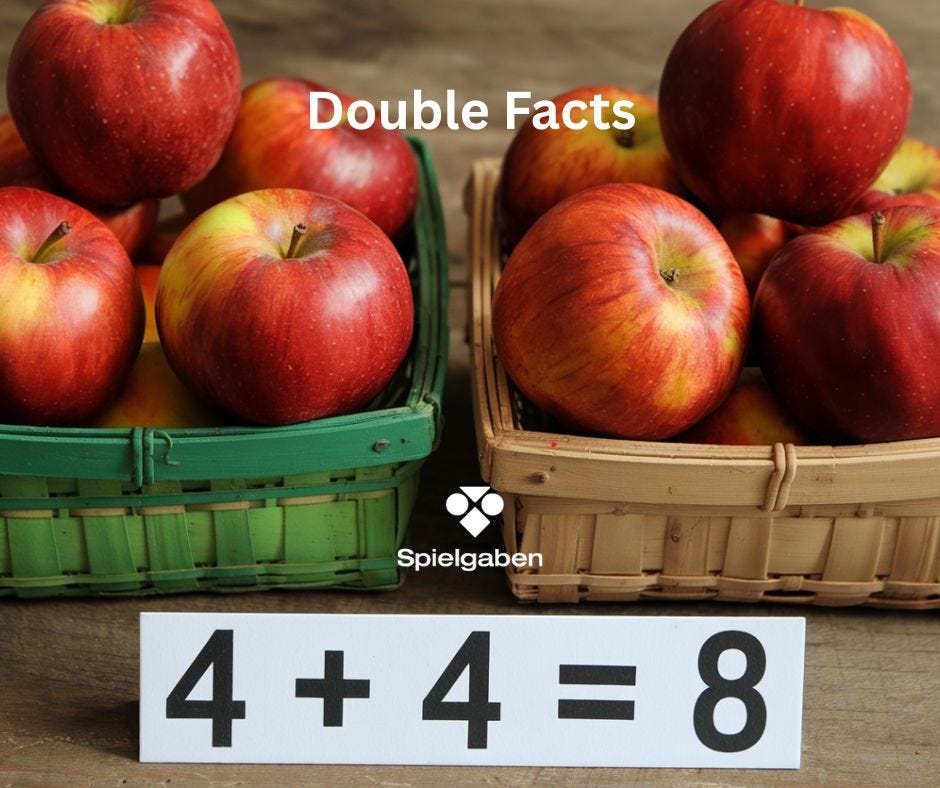
Subject: Doubles Facts (2+2, 3+3, etc.)

1. Alignment with Standards:

• CCSS.MATH.CONTENT.1.OA.C.6
  • Add and subtract within 20, demonstrating fluency for addition and subtraction within 10.
• CCSS.MATH.CONTENT.1.OA.B.3
  • Apply properties of operations as strategies to add and subtract.

2. Lesson Objectives

By the end of this lesson, children will be able to:

• Identify and recite doubles addition facts (1+1 through 10+10).
• Use manipulatives (counters, dominoes, etc.) to model doubles.
• Solve simple word problems involving doubles.

3. Materials Needed

• Counters (buttons, beads, or small blocks)
• Doubles Flashcards (with visuals like dots or fingers)
• Printable Doubles Worksheet (with pictures and equations)
• Dominoes (to find doubles)
• Whiteboard & markers
• Image Prompt (Visual aid showing doubles, e.g., two sets of 3 apples)

4. Lesson Activities

A. Warm-Up (5-10 min): Counting by Twos

• Sing a “Counting by Twos” song (e.g., 2, 4, 6, 8, 10…) to introduce the concept of doubling.
• Ask: “If I have 2 cookies and you have 2 cookies, how many do we have together?”

B. Direct Instruction (10 min): Introducing Doubles

• Show the image prompt (e.g., two groups of 3 stars).
• Write the equation 3 + 3 = 6 and explain: “When we add the same number twice, it’s called a double!”
• Demonstrate with counters:
  • Place 2 counters + 2 counters, then count all to get 4.
  • Repeat with 4 + 4, 5 + 5, etc.

C. Guided Practice (15 min): Hands-On Activities

1. Domino Doubles Match
   • Lay out dominoes face up. Have the student find dominoes with the same number on both sides (e.g., 4-4).
   • Say: *”This is 4 + 4 = 8!”*
2. Doubles Flashcards Drill
   • Show flashcards with visuals (e.g., two sets of 5 dots) and ask for the total.
3. Whiteboard Practice
   • Write a doubles fact (e.g., 6 + 6) and have the student solve it using counters.

D. Independent Practice (10 min): Worksheet & Game

• Worksheet: Solve doubles problems with picture support (e.g., two buses with 5 kids each).
• Roll & Double Game:
  • Roll a die, then double the number (e.g., roll a 3 → 3 + 3 = 6).

E. Wrap-Up (5 min): Real-Life Doubles

• Ask: “Can you think of real-life doubles?” (e.g., two eyes, two hands, two legs).
• Quick verbal quiz: *”What’s 7 + 7?”*

5. Assessment

• Observation: Can the student quickly recall doubles up to 10+10?
• Worksheet Accuracy: Check for correct answers.
• Exit Question: *”What is 8 + 8?”*

6. Extension Ideas

• Doubles +1: Introduce near-doubles (e.g., *If 5+5=10, then 5+6=11*).
• Doubles Bingo: Create a bingo game with doubles sums.

Mid Level (Grade 3 to 5)

Subject: Adding and Subtracting Fractions with Like Denominators

1. Alignment with Standards:

• CCSS.MATH.CONTENT.4.NF.B.3.A
  • Understand addition and subtraction of fractions as joining and separating parts referring to the same whole.
• CCSS.MATH.CONTENT.4.NF.B.3.D
  • Solve word problems involving addition and subtraction of fractions with like denominators.

2. Lesson Objectives

Children will be able to:

• Add and subtract fractions with like denominators using visual models (fraction bars, circles).
• Solve problems and simplify fractions when possible (e.g., 2/8 → 1/4).
• Apply skills to real-world scenarios (e.g., pizza slices, measuring ingredients).

3. Materials Needed

• Fraction Bars or Circles (physical or printable)
• Whiteboard & Markers
• Colored Pencils & Grid Paper (for shading fractions)
• Word Problem Cards (real-life examples)
• Image Prompt (e.g., pizza divided into eighths with slices added/subtracted)

4. Lesson Activities

A. Warm-Up (5-10 min): Fraction Review

• Ask: *”If I eat 1/4 of a sandwich and then another 1/4, how much did I eat total?”*
• Draw a fraction bar divided into fourths to visually confirm 2/4 = 1/2.

B. Direct Instruction (15 min): Concept & Examples

1. Key Rule: “When denominators are the same, add/subtract the numerators and keep the denominator.”
   • Example: 3/6 + 2/6 = 5/6 (show with fraction circles).
2. Simplifying: *”Can we make 4/8 smaller?”* → Fold fraction bars to show 4/8 = 1/2.

C. Guided Practice (20 min): Hands-On Learning

1. Fraction Bar Manipulatives
   • Students solve 5/8 – 3/8 by removing shaded sections.
2. Number Line Jumping
   • Plot 1/5, then add 2/5 → *”We land on 3/5!”*
3. Word Problems
   • *”A recipe needs 3/4 cup of sugar. You add 1/4 cup. How much is there now?”*

D. Independent Practice (15 min): Worksheets & Games

• Worksheet: Solve equations like 7/10 – 4/10 and shade grids to match.
• Fraction War Card Game:
  • Draw two fractions with like denominators (e.g., 3/5 and 4/5), add/subtract, and compare results.

E. Wrap-Up (5 min): Real-World Connection

• Discuss: “When might you add/subtract fractions in life?” (e.g., measuring wood, sharing food).
• Exit Ticket: Solve 2/3 + 1/3 and 9/12 – 5/12, then simplify if possible.

5. Assessment

• Observation: Can the student explain the steps while using manipulatives?
• Worksheet Accuracy: Check for correct operations and simplification.
• Exit Ticket Responses: Quick verification of understanding.

6. Extension Ideas

• Mixed Numbers: Introduce problems like 1 3/5 + 2/5.
• Error Analysis: Provide a solved problem with a mistake (e.g., 2/7 + 3/7 = 5/14) and ask students to find the error.

High Level (Grade 6 to 8)

Subject: Solving One-Step Equations

1. Alignment with Standards:

• CCSS.MATH.CONTENT.7.EE.B.4.A
  • Solve real-world problems leading to equations of the form px + q = r and p(x + q) = r, where *p*, *q*, and *r* are rational numbers.
• CCSS.MATH.CONTENT.7.EE.B.3
  • Solve multi-step problems with positive and negative rational numbers in any form.

2. Lesson Objectives

Children will be able to:

• Identify inverse operations (e.g., subtraction “undoes” addition).
• Solve one-step equations involving integers, fractions, and decimals.
• Justify solutions by checking answers in the original equation.
• Apply skills to word problems (e.g., “A number divided by 5 equals 3”).

3. Materials Needed

• Algebra Tiles (or colored paper squares for visual modeling)
• Whiteboard & Markers
• Equation Cards (for sorting and solving)
• Real-World Problem Strips (e.g., shopping discounts, speed calculations)
• Image Prompt (Balance scale showing *x + 2 = 5*)

4. Lesson Activities

A. Warm-Up (10 min): Inverse Operations Review

• Think-Pair-Share: “How would you undo these operations?”
  • Example: “If I add 7, how do I reverse it?” (Subtract 7)
• Quick Practice: Solve mentally: *x – 4 = 10*; *3n = 15*.

B. Direct Instruction (20 min): Key Concepts & Examples

1. Introduce Inverse Operations:
   • Addition ↔ Subtraction | Multiplication ↔ Division
   • Demonstrate with algebra tiles: *x + 3 = 7* → Remove 3 tiles from both sides.
2. Solve Equations Step-by-Step:
   • Example 1: *m – 5 = 12* (Add 5 to both sides).
   • Example 2: *x/4 = 6* (Multiply both sides by 4).
3. Check Solutions: Substitute back (e.g., *17 – 5 = 12* ✓).

C. Guided Practice (25 min): Hands-On Learning

1. Algebra Tile Exploration:
   • Model *2x = 8* by dividing tiles into two equal groups.
2. Equation Sorting:
   • Sort cards into “Add/Subtract” or “Multiply/Divide” categories before solving.
3. Error Analysis:
   • “Spot the Mistake”: Provide incorrect solutions (e.g., *x + 9 = 11 → x = 20*).

D. Independent Practice (20 min): Worksheets & Real-World Tasks

• Tiered Worksheets:
  • Level 1: *y + 8 = 15*
  • Level 2: *−5 = a/3*
  • Level 3: Word problems (e.g., “A number tripled is 27”).
• Speed Challenge: Timed solve-and-check with partner.

E. Wrap-Up (10 min): Reflection & Application

• Real-World Discussion: “Why is solving equations useful?” (e.g., budgeting, cooking).
• Exit Ticket: Solve k – 6 = −4, and 7 x = 49, then explain steps.

5. Assessment

• Participation: Observes use of algebra tiles and verbal reasoning.
• Worksheet Accuracy: Tracks correct application of inverse operations.
• Exit Ticket: Evaluates procedural and conceptual understanding.

6. Extension Ideas

• Negative Coefficients: Solve equations like *−x = 9*.
• Two-Step Preview: Introduce *2x + 1 = 7* for advanced learners.
• Create Your Own: Students design word problems for peers to solve.